2023 AIChE Annual Meeting
(373a) Integrated Metabolic and Compartment Model for Simulations of Large-Scale Multi-Impeller Aerated Bioreactors
Authors
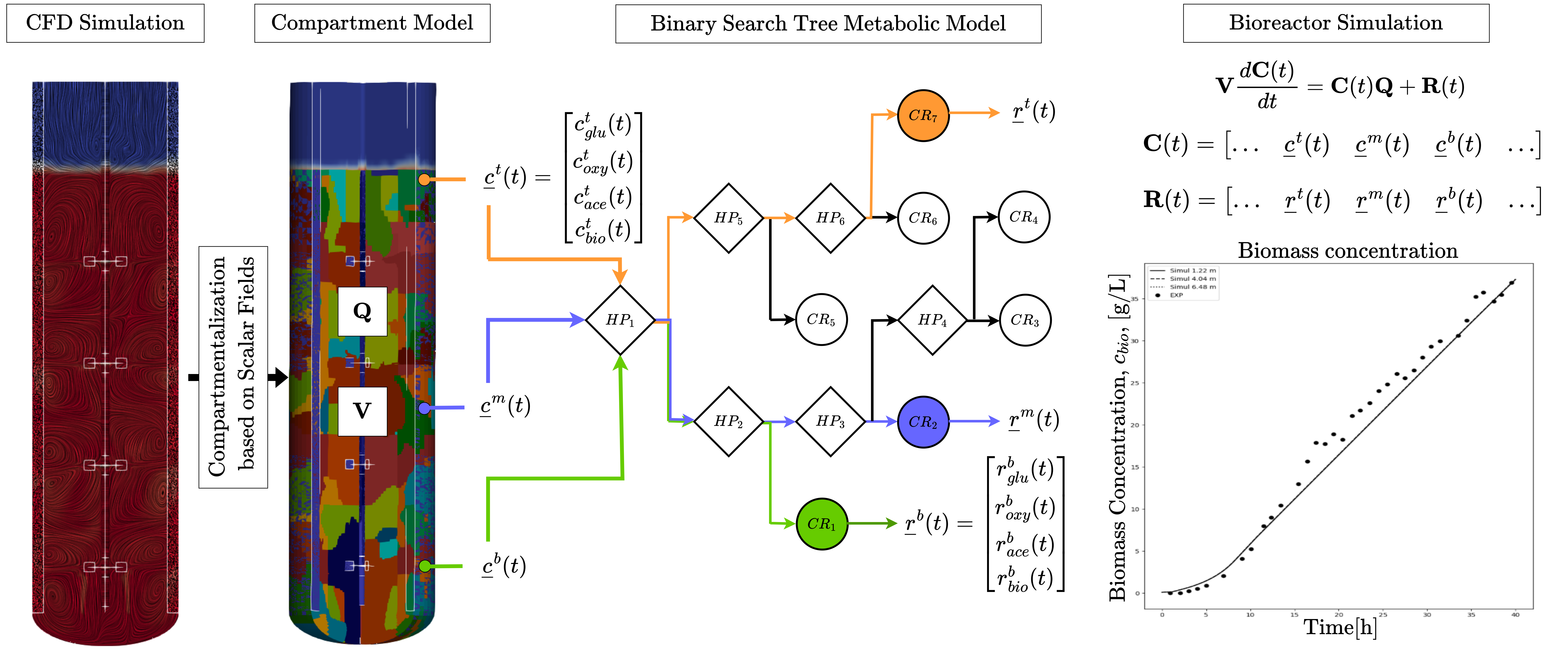
A multi-parametric solver, MPT3, was used to produce critical regions expressing analytical equations for flux computation for each active set of DFBM constraints. After identifying the critical regions corresponding to each active set of constraints, the LP problem is then converted into a parametric point location problem. The data structure encoding the map (i.e. hash table, BST, k-dimensional tree (KDT), etc.) is then developed and its related point location method employed to accelerate the search for the optimal solution. This method has been frequently applied in the field of explicit model predictive control, where it displays outstanding online computation time reduction, depending on the point location method and data structure chosen. The BST data structure was chosen due to its very efficient online searching time scaling of O(log n), with n being the number of items. The BST structure is based on division of the critical region map using hyperplanes (HP) between each of the critical regions. A sample BST corresponding to a critical region map is shown the Figure. The point location algorithm is then used to determine the path through the tree to reach the leaf node, for which an analytical expression for the DFBM is uniquely defined. Search through the BST is based on determined the side of the hyperplane (in the parameter space) where the concentration vector (c) is located. Once the leaf node is identifed, the reaction rate vector (r) predicted by the DFBM is computed through a simple evaluation of an analytical expression.
As most reported compartmental models are based on a velocity field of the two-dimensional section of the stirred tank, the azimuthal (tangential) components have not taken into account in reported studies. This results in inaccuracies in the corresponding compartmental model, due to unsteady three-dimensional nature of turbulent multiphase flows. In this work, the compartmentalization approach is informed by fully three-dimensional velocity fields resulting from CFD simulation to improve accuracy and fidelity. A compartmentalization algorithm based on scalar fields is applied to both decompose the domain into compartments, compute net volumetric flow rates between compartments (Q), and active volume for each compartment (V). An example of this process is shown in the Figure, along with the resulting compartmental model equations.
In order to validate the presented methodology, a BSTMM of E. coli is combined with the compartment model obtained from a CFD simulation of a 20 cubic meter four-impeller aerated bioreactor. The BSTMM was developed, and then the coefficients of the model were calibrated using experimental data of E. coli in batch operation of a 7 liter bioreactor reported in the literature. The calibrated model was applied for prediction of experimental concentrations obtained in fed-batch operation and compared to those reported in the literature. The model results are in good agreement with concentration profiles reported from the experiments. The simulation is able to predict gradients of concentrations, variations in reaction rates and cell growth limits in different zones of the bioreactor. Thus, the proposed approach provides a better understanding of the impacts of heterogeneity inside the bioreactor on a microbial population.
The integrated metabolic/compartment model presented is shown to enable the simulation of large-scale bioreactors over industrially relevant time-scales without significant loss of accuracy. While it requires accurate CFD simulations for compartmentalization and a calibrated BSTMM, it enables computationally efficient simulations which would be infeasible with an integrated CFD/DFBM method.