2020 Virtual AIChE Annual Meeting
(3bj) Multicomponent Diffusion of Interacting, Nonionic Micelles with Hydrophobic Solutes
Authors
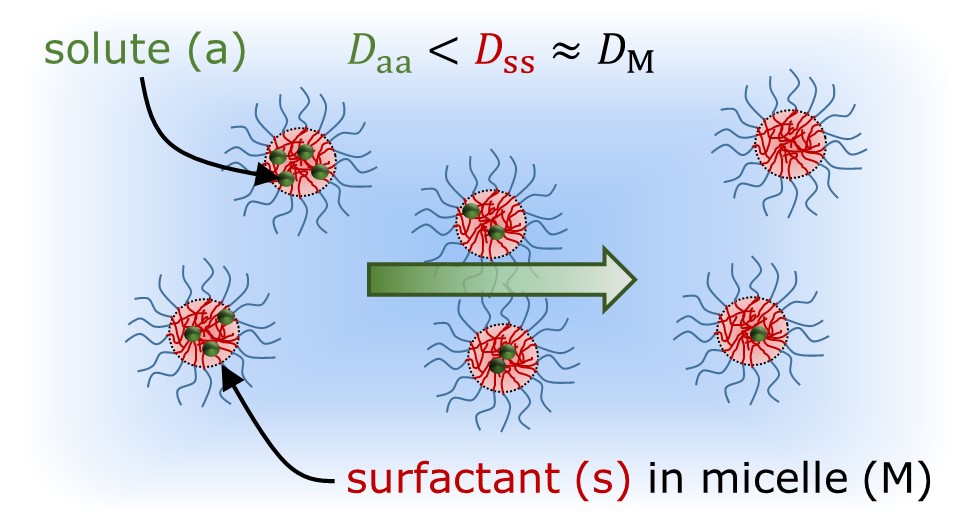
Ternary diffusion in solutions of nonionic surfactants and hydrophobic solutes is particularly interesting because strong solute and surfactant interactions persist across all micelle concentrations, from dilute to crowded conditions. In this work, Taylor dispersion and dynamic light scattering techniques were used to measure the ternary diffusion coefficient matrix [D], and eigenvalues D+ and D-, in crowded aqueous solutions of decaethylene glycol monododecyl ether (C12E10) with either decane or limonene. The results at low solute to surfactant molar ratios indicate that C12E10 and hydrophobic solute diffused down their respective gradients with diffusivities similar to the fast D+ and slow D- eigenvalues, respectively. Furthermore, strong diffusion coupling, comprising solute diffusion down a surfactant gradient and surfactant diffusion up a solute gradient, was also observed, with cross diffusivities that were on the order of or larger than the main diffusivities. Measurements of the average micelle aggregation number, hydration index, and hydrodynamic radius, obtained using both static and dynamic light scattering methods, indicate that solute-containing micelles interacted as hard spheres with an average radius and aggregation number that increased linearly with the molar ratio of solute to surfactant for both hydrophobic solutes. A theoretical model, developed using Batchelorâs theory for gradient diffusion in a polydisperse system of interacting hard spheres, was effectively used to predict [D], D+, and D- with no adjustable parameters. Comparison with theory indicates the eigenvalues D- and D+ correspond to longâtime self and gradient diffusion for monodisperse hard spheres.